Atmospheric Electricity. 1. It was not until the middle of the 18th century that experiments due to Benjamin Franklin showed that the electric phenomena of the atmosphere are not fundamentally different from those produced in the laboratory. For the next century the rate of progress was slow, though the ideas of Volta in Italy and the instrumental devices of Sir Francis Ronalds in England merit recognition. The invention of the portable electrometer and the water-dropping electrograph by Lord Kelvin in the middle of the 19th century, and the greater definiteness thus introduced into observational results, were notable events. Towards the end of the 19th century came the discovery made by W. Linss (6)1 and by J. Elster and H. Geitel (7) that even the most perfectly insulated conductors lose their charge, and that this loss depends on atmospheric conditions. Hard on this came the recognition of the fact that freely charged positive and negative ions are always present in the atmosphere, and that a radioactive emanation can be collected. Whilst no small amount of observational work has been done in these new branches of atmospheric electricity, the science has still not developed to a considerable extent beyond preliminary stages. Observations have usually been limited to a portion of the year, or to a few hours of the day, whilst the results from different stations differ much in details. It is thus difficult to form a judgment as to what has most claim to acceptance as the general law, and what may be regarded as local or exceptional.
2. Potential Gradient.—In dry weather the electric potential in the atmosphere is normally positive relative to the earth, and increases with the height. The existence of earth currents (q.v.) shows that the earth, strictly speaking, is not all at one potential, but the natural differences of potential between points on the earth’s surface a mile apart are insignificant compared to the normal potential difference between the earth and a point one foot above it. What is aimed at in ordinary observations of atmospheric potential is the measurement of the difference of potential between the earth and a point a given distance above it, or of the difference of potential between two points in the same vertical line a given distance apart. Let a conductor, say a metallic sphere, be supported by a metal rod of negligible electric capacity whose other end is earthed. As the whole conductor must be at zero (i.e. the earth’s) potential, there must be an induced charge on the sphere, producing at its centre a potential equal but of opposite sign to what would exist at the same spot in free air. This neglects any charge in the air displaced by the sphere, and assumes a statical state of conditions and that the conductor itself exerts no disturbing influence. Suppose now that the sphere’s earth connexion is broken and that it is carried without loss of charge inside a building at zero potential. If its potential as observed there is −V (volts), then the potential of the air at the spot occupied by the sphere was +V. This method in one shape or another has been often employed. Suppose next that a fixed insulated conductor is somehow kept at the potential of the air at a given point, then the measurement of its potential is equivalent to a measurement of that of the air. This is the basis of a variety of methods. In the earliest the conductor was represented by long metal wires, supported by silk or other insulating material, and left to pick up the air’s potential. The addition of sharp points was a step in advance; but the method hardly became a quantitative one until the sharp points were replaced by a flame (fuse, gas, lamp), or by a liquid jet breaking into drops. The matter leaving the conductor, whether the products of combustion or the drops of a liquid, supplies the means of securing equality of potential between the conductor and the air at the spot where the matter quits electrical connexion with the conductor. Of late years the function of the collector is discharged in some forms of apparatus by a salt of radium. Of flame collectors the two best known are Lord Kelvin’s portable electrometer with a fuse, or F. Exner’s gold leaf electroscope in conjunction with an oil lamp or gas flame. Of liquid collectors the representative is Lord Kelvin’s water-dropping electrograph; while Benndorf’s is the form of radium collector that has been most used. It cannot be said that any one form of collector is superior all round. Flame collectors blow out in high winds, whilst water-droppers are apt to get frozen in winter. At first sight the balance of advantages seems to lie with radium. But while gaseous products and even falling water are capable of modifying electrical conditions in their immediate neighbourhood, the “infection” produced by radium is more insidious, and other drawbacks present themselves in practice. It requires a radium salt of high radioactivity to be at all comparable in effectiveness with a good water-dropper. Experiments by F. Linke (8) indicated that a water-dropper having a number of fine holes, or having a fine jet under a considerable pressure, picks up the potential in about a tenth of the time required by the ordinary radium preparation protected by a glass tube. These fine jet droppers with a mixture of alcohol and water have proved very effective for balloon observations.
Table I.—Annual Variation Potential Gradient.
| Place and Period. | Jan. | Feb. | March. | April. | May. | June. | July. | Aug. | Sept. | Oct. | Nov. | Dec. |
| Karasjok (10), 1903-1904 | 143 | 150 | 137 | 94 | 74 | 65 | 70 | 67 | 67 | 87 | 120 | 126 |
| Sodankylä (31), 1882-1883 | 94 | 133 | 148 | 155 | 186 | 93 | 53 | 77 | 47 | 72 | 71 | 71 |
| Potsdam (9), 1904 | 167 | 95 | 118 | 88 | 93 | 72 | 73 | 65 | 97 | 101 | 108 | 123 |
| Kew (12), 1898-1904 | 127 | 141 | 113 | 87 | 77 | 70 | 61 | 72 | 76 | 96 | 126 | 153 |
| Greenwich (13), 1893-1894, 1896 | 110 | 112 | 127 | 107 | 83 | 71 | 76 | 84 | 83 | 104 | 104 | 139 |
| Florence (14), 1883-1886 | 132 | 110 | 98 | 84 | 86 | 81 | 77 | 90 | 89 | 99 | 129 | 125 |
| Perpignan (15), 1886-1888 | 121 | 112 | 108 | 89 | 91 | 92 | 89 | 82 | 74 | 99 | 122 | 121 |
| Lisbon (16), 1884-1886 | 104 | 105 | 104 | 92 | 91 | 93 | 87 | 92 | 100 | 99 | 115 | 117 |
| Tokyo (17), 1897-1898, 1900-1901 | 165 | 145 | 117 | 86 | 62 | 58 | 41 | 59 | 59 | 97 | 134 | 176 |
| Batavia (18)(2 m.), 1887-1890 | 97 | 115 | 155 | 127 | 129 | 105 | 79 | 62 | 69 | 79 | 90 | 93 |
| Batavia (7.8 m.) 1890-1895 | 100 | 89 | 103 | 120 | 98 | 103 | 85 | 99 | 73 | 101 | 117 | 112 |
3. Before considering observational data, it is expedient to mention various sources of uncertainty. Above the level plain of absolutely smooth surface, devoid of houses or vegetation, the equipotential surfaces under normal conditions would be strictly horizontal, and if we could determine the potential at one metre above the ground we should have a definite measure of the potential gradient at the earth’s surface. The presence, however, of apparatus or observers upsets the conditions, while above uneven ground or near a tree or a building the equipotential surfaces cease to be horizontal. In an ordinary climate a building seems to be practically at the earth’s potential; near its walls the equipotential surfaces are highly inclined, and near the ridges they may lie very close together. The height of the walls in the various observatories, the height of the collectors, and the distance they project from the wall vary largely, and sometimes there are external buildings or trees sufficiently near to influence the potential. It is thus futile to compare the absolute voltages met with at two stations, unless allowance can be made for the influence of the environment. With a view to this, it has become increasingly common of late years to publish not the voltages actually observed, but values deduced from them for the potential gradient in the open in volts per metre. Observations are made at a given height over level open ground near the observatory, and a comparison with the simultaneous results from the self-recording electrograph enables the records from the latter to be expressed as potential gradients in the open. In the case, however, of many observatories, especially as regards the older records, no data for reduction exist; further, the reduction to the open is at best only an approximation, the success attending which probably varies considerably at different stations. This is one of the reasons why in the figures for the annual and diurnal variations in Tables I., II. and III., the potential has been expressed as percentages of its mean value for the year or the day. In most cases the environment of a collector is not absolutely invariable. If the shape of the equipotential surfaces near it is influenced by trees, shrubs or grass, their influence will vary throughout the year. In winter the varying depth of snow may exert an appreciable effect. There are sources of uncertainty in the instrument itself. Unless the insulation is perfect, the potential recorded falls short of that at the spot where the radium is placed or the water jet breaks. The action of the collector is opposed by the leakage through imperfect insulation, or natural dissipation, and this may introduce a fictitious element into the apparent annual or diurnal variation. The potentials that have to be dealt with are often hundreds and sometimes thousands of volts, and insulation troubles are more serious than is generally appreciated. When a water jet serves as collector, the pressure under which it issues should be practically constant. If the pressure alters as the water tank empties, a discontinuity occurs in the trace when the tank is refilled, and a fictitious element may be introduced into the diurnal variation. When rain or snow is falling, the potential frequently changes rapidly. These changes are often too rapid to be satisfactorily dealt with by an ordinary electrometer, and they sometimes leave hardly a trace on the photographic paper. Again rain dripping from exposed parts of the apparatus may materially affect the record. It is thus customary in calculating diurnal inequalities either to take no account of days on which there is an appreciable rainfall, or else to form separate tables for “dry” or “fine” days and for “all” days. Speaking generally, the exclusion of days of rain and of negative potential comes pretty much to the same thing, and the presence or absence of negative potential is not infrequently the criterion by reference to which days are rejected or are accepted as normal.
4. The potential gradient near the ground varies with the season of the year and the hour of the day, and is largely dependent on the weather conditions. It is thus difficult to form even a rough estimate of the mean value at any place unless hourly readings exist, extending over the whole or the greater part of a year. It is even somewhat precipitate to assume that a mean value deduced from a single year is fairly representative of average conditions. At Potsdam, G. Lüdeling (9) found for the mean value for 1904 in volts per metre 242. At Karasjok in the extreme north of Norway G.C. Simpson (10) in 1903-1904 obtained 139. At Kremsmünster for 1902 P.B. Zölss(11) gives 98. At Kew (12) the mean for individual years from 1898 to 1904 varied from 141 in 1900 to 179 in 1899, the mean from the seven years combined being 159. The large difference between the means obtained at Potsdam and Kremsmünster, as compared to the comparative similarity between the results for Kew and Karasjok, suggests that the mean value of the potential gradient may be much more dependent on local conditions than on difference of latitude.
At any single station potential gradient has a wide range of values. The largest positive and negative values recorded are met with during disturbed weather. During thunderstorms the record from an electrograph shows large sudden excursions, the trace usually going off the sheet with every flash of lightning when the thunder is near. Exactly what the potential changes amount to under such circumstances it is impossible to say; what the trace shows depends largely on the type of electrometer. Large rapid changes are also met with in the absence of thunder during heavy rain or snow fall. In England the largest values of a sufficiently steady character to be shown correctly by an ordinary electrograph occur during winter fogs. At such times gradients of +400 or +500 volts per metre are by no means unusual at Kew, and voltages of 700 or 800 are occasionally met with.
5. Annual Variation.—Table I. gives the annual variation of the potential gradient at a number of stations arranged according to latitude, the mean value for the whole year being taken in each case as 100. Karasjok as already mentioned is in the extreme north of Norway (69° 17′ N.); Sodankylä was the Finnish station of the international polar year 1882-1883. At Batavia, which is near the equator (6° 11′ S.) the annual variation seems somewhat irregular. Further, the results obtained with the water-dropper at two heights—viz. 2 and 7.8 metres—differ notably. At all the other stalions the difference between summer and winter months is conspicuous. From the European data one would be disposed to conclude that the variation throughout the year diminishes as one approaches the equator. It is decidedly less at Perpignan and Lisbon than at Potsdam, Kew and Greenwich, but nowhere is the seasonal difference more conspicuous than at Tokyo, which is south of Lisbon.
Table II.—Diurnal Variation Potential Gradient.
| Station. | Karasjok. | Sodankylä. | Kew(19, 12). | Greenwich. | Florence. | Perpignan. | Lisbon. | Tokyo. | Batavia. | Cape Horn(20). |
||
| Period. | 1903-4. | 1882-83. | 1862- 1864. |
1898- 1904. |
1893-96. | 1883-85. | 1886-88. | 1884-86. | 1897-98 1900-1. |
1887- 1890. |
1890- 1895. |
1882-83. |
| Days. | All. | All. | Quiet. | All. | All. | Fine. | All. | All. | Dry. | Dry. | Pos. | |
| h l |
5.5 | 3.0 2.5 |
3.5 1.0 |
3.35 1.3 |
3.0 1.8 |
8.4 1.5 |
3.0 0.5 |
1.7 2.0 |
2 | 7.8 | 3.5 2.0 |
|
| Hour. | ||||||||||||
| 1 | 83 | 91 | 87 | 93 | 97 | 92 | 78 | 84 | 101 | 147 | 125 | 82 |
| 2 | 73 | 85 | 79 | 88 | 89 | 83 | 72 | 80 | 98 | 141 | 114 | 73 |
| 3 | 66 | 82 | 74 | 84 | 87 | 77 | 71 | 78 | 97 | 135 | 109 | 85 |
| 4 | 63 | 84 | 72 | 83 | 86 | 75 | 72 | 81 | 99 | 128 | 102 | 81 |
| 5 | 60 | 89 | 71 | 85 | 86 | 74 | 77 | 83 | 121 | 127 | 101 | 85 |
| 6 | 68 | 91 | 77 | 93 | 92 | 82 | 92 | 92 | 154 | 137 | 117 | 95 |
| 7 | 81 | 97 | 92 | 103 | 100 | 100 | 107 | 101 | 167 | 158 | 147 | 106 |
| 8 | 87 | 100 | 106 | 112 | 102 | 112 | 114 | 105 | 149 | 104 | 119 | 118 |
| 9 | 94 | 98 | 107 | 115 | 100 | 113 | 111 | 104 | 117 | 67 | 82 | 119 |
| 10 | 101 | 102 | 100 | 112 | 101 | 107 | 100 | 104 | 87 | 42 | 55 | 123 |
| 11 | 99 | 98 | 90 | 101 | 96 | 100 | 96 | 102 | 70 | 35 | 46 | 123 |
| Noon. | 103 | 102 | 92 | 94 | 97 | 95 | 99 | 108 | 61 | 30 | 43 | 115 |
| 1 | 106 | 105 | 90 | 89 | 96 | 92 | 99 | 111 | 54 | 30 | 42 | 112 |
| 2 | 108 | 107 | 91 | 87 | 94 | 90 | 97 | 114 | 49 | 30 | 43 | 94 |
| 3 | 108 | 108 | 92 | 88 | 95 | 89 | 99 | 109 | 53 | 33 | 46 | 89 |
| 4 | 109 | 108 | 98 | 93 | 97 | 89 | 105 | 108 | 61 | 41 | 53 | 88 |
| 5 | 110 | 108 | 108 | 99 | 102 | 94 | 113 | 108 | 76 | 67 | 73 | 84 |
| 6 | 119 | 110 | 121 | 108 | 108 | 113 | 126 | 111 | 95 | 91 | 108 | 110 |
| 7 | 129 | 102 | 134 | 115 | 111 | 121 | 131 | 116 | 107 | 120 | 145 | 107 |
| 8 | 136 | 111 | 139 | 118 | 115 | 129 | 129 | 114 | 114 | 137 | 155 | 123 |
| 9 | 139 | 111 | 138 | 119 | 117 | 132 | 120 | 109 | 119 | 146 | 155 | 112 |
| 10 | 133 | 104 | 128 | 115 | 117 | 127 | 109 | 102 | 120 | 148 | 147 | 99 |
| 11 | 121 | 108 | 113 | 108 | 111 | 114 | 97 | 92 | 119 | 151 | 143 | 85 |
| 12 | 102 | 93 | 99 | 99 | 104 | 100 | 86 | 85 | 112 | 147 | 130 | 98 |
Table III.—Diurnal Variation Potential Gradient.
| Station. | Karasjok. | Sodankylä. | Kew. | Greenwich. | Bureau Central (21). |
Eiffel Tower (21). |
Perpignan (21). | Batavia. (2 m.) |
||||||||
| Period. | 1903-4. | 1882-83. | 1898-1904. | 1894 and ’96. | 1894-99. | 1896-98. | 1885-95. | 1887-90. | ||||||||
| Winter. | Summer. | Winter. | Summer. | Winter. | Equinox. | Summer. | Winter. | Summer. | Winter. | Summer. | Summer. | Winter. | Summer. | Winter. | Summer. | |
| Hour. | ||||||||||||||||
| 1 | 76 | 104 | 90 | 99 | 91 | 93 | 96 | 87 | 110 | 79 | 102 | 90 | 72 | 88 | 145 | 149 |
| 2 | 66 | 96 | 79 | 84 | 86 | 88 | 90 | 84 | 101 | 71 | 92 | 83 | 67 | 83 | 139 | 142 |
| 3 | 57 | 89 | 78 | 90 | 82 | 85 | 85 | 76 | 98 | 70 | 88 | 79 | 66 | 81 | 137 | 135 |
| 4 | 55 | 83 | 74 | 99 | 81 | 84 | 84 | 77 | 96 | 69 | 84 | 76 | 67 | 83 | 131 | 127 |
| 5 | 50 | 79 | 74 | 111 | 82 | 87 | 90 | 78 | 94 | 75 | 94 | 78 | 72 | 92 | 132 | 123 |
| 6 | 61 | 83 | 80 | 114 | 86 | 97 | 101 | 82 | 101 | 83 | 106 | 87 | 84 | 107 | 138 | 136 |
| 7 | 78 | 89 | 86 | 117 | 95 | 109 | 113 | 94 | 107 | 98 | 118 | 97 | 104 | 114 | 166 | 153 |
| 8 | 82 | 93 | 95 | 122 | 104 | 118 | 120 | 97 | 111 | 111 | 120 | 103 | 122 | 108 | 118 | 92 |
| 9 | 90 | 93 | 91 | 109 | 111 | 119 | 119 | 98 | 102 | 113 | 106 | 110 | 126 | 100 | 74 | 64 |
| 10 | 104 | 93 | 106 | 101 | 114 | 110 | 110 | 102 | 98 | 111 | 94 | 109 | 114 | 93 | 43 | 40 |
| 11 | 102 | 92 | 98 | 97 | 107 | 95 | 97 | 103 | 86 | 108 | 84 | 107 | 98 | 90 | 35 | 36 |
| Noon. | 119 | 90 | 98 | 100 | 102 | 86 | 87 | 107 | 94 | 106 | 77 | 104 | 99 | 95 | 31 | 30 |
| 1 | 116 | 94 | 116 | 97 | 99 | 81 | 80 | 107 | 85 | 112 | 79 | 107 | 96 | 93 | 29 | 33 |
| 2 | 118 | 97 | 113 | 97 | 97 | 80 | 76 | 109 | 82 | 112 | 81 | 110 | 94 | 90 | 28 | 32 |
| 3 | 119 | 100 | 121 | 93 | 99 | 82 | 76 | 111 | 78 | 111 | 78 | 107 | 95 | 88 | 24 | 41 |
| 4 | 115 | 99 | 111 | 96 | 103 | 88 | 80 | 116 | 81 | 113 | 80 | 105 | 102 | 92 | 30 | 49 |
| 5 | 120 | 106 | 105 | 106 | 108 | 96 | 87 | 112 | 93 | 120 | 85 | 106 | 115 | 98 | 60 | 74 |
| 6 | 131 | 104 | 115 | 92 | 111 | 109 | 98 | 114 | 98 | 124 | 97 | 109 | 128 | 110 | 88 | 94 |
| 7 | 136 | 110 | 118 | 102 | 114 | 120 | 111 | 117 | 99 | 124 | 123 | 113 | 133 | 122 | 119 | 122 |
| 8 | 134 | 113 | 117 | 106 | 112 | 124 | 123 | 113 | 108 | 116 | 134 | 110 | 131 | 127 | 138 | 135 |
| 9 | 137 | 125 | 115 | 90 | 111 | 123 | 129 | 111 | 118 | 104 | 130 | 109 | 124 | 125 | 145 | 147 |
| 10 | 125 | 135 | 112 | 90 | 108 | 118 | 125 | 110 | 124 | 97 | 122 | 105 | 111 | 117 | 148 | 148 |
| 11 | 114 | 126 | 113 | 103 | 103 | 109 | 116 | 102 | 120 | 90 | 115 | 101 | 96 | 108 | 149 | 152 |
| 12 | 96 | 111 | 95 | 85 | 96 | 99 | 105 | 93 | 116 | 83 | 108 | 94 | 83 | 95 | 148 | 146 |
At the temperate stations the maximum occurs near midwinter; in the Arctic it seems deferred towards spring.
6. Diurnal Variation.—Table II. gives the mean diurnal variation for the whole year at a number of stations arranged in order of latitude, the mean from the 24 hourly values being taken as 100. The data are some from “all” days, some from “quiet,” “fine” or “dry” days. The height, h, and the distance from the wall, l, were the potential is measured are given in metres when known. In most cases two distinct maxima and minima occur in the 24 hours. The principal maximum is usually found in the evening between 8 and 10 P.M., the principal minimum in the morning from 3 to 5 A.M. At some stations the minimum in the afternoon is indistinctly shown, but at Tokyo and Batavia it is much more conspicuous than the morning minimum.
 |
7. In Table III. the diurnal inequality is shown for “winter” and “summer” respectively. In all cases the mean value for the 24 hours is taken as 100. By “summer” is meant April to September at Sodankylä, Greenwich and Batavia; May to August at Kew, Bureau Central (Paris), Eiffel Tower and Perpignan; and May to July at Karasjok. “Winter” includes October to March at Sodankylä, Greenwich and Batavia; November to February at Kew and Bureau Central; November to January at Karasjok, and December and January at Perpignan. Mean results from March, April, September and October at Kew are assigned to “Equinox.”
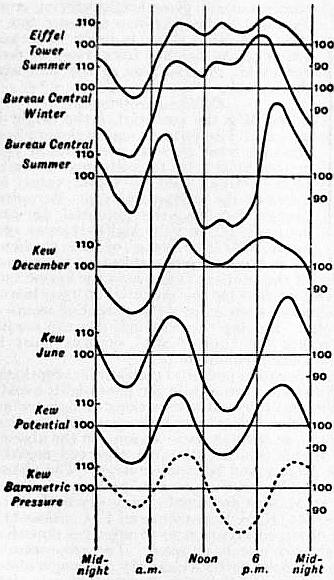
At Batavia the difference between winter and summer is comparatively small. Elsewhere there is a tendency for the double period, usually so prominent in summer, to become less pronounced in winter, the afternoon minimum tending to disappear. Even in summer the double period is not prominent in the arctic climate of Karasjok or on the top of the Eiffel Tower. The diurnal variation in summer at the latter station is shown graphically in the top curve of fig. 1. It presents a remarkable resemblance to the adjacent curve, which gives the diurnal variation at mid-winter at the Bureau Central. The resemblance between these curves is much closer than that between the Bureau Central’s own winter and summer curves. All three Paris curves show three peaks, the first and third representing the ordinary forenoon and afternoon maxima. In summer at the Bureau Central the intermediate peak nearly disappears in the profound afternoon depression, but it is still recognizable. This three-peaked curve is not wholly peculiar to Paris, being seen, for instance, at Lisbon in summer. The December and June curves for Kew are good examples of the ordinary nature of the difference between midwinter and midsummer. The afternoon minimum at Kew gradually deepens as midsummer approaches. Simultaneously the forenoon maximum occurs earlier and the afternoon maximum later in the day. The two last curves in the diagram contrast the diurnal variation at Kew in potential gradient and in barometric pressure for the year as a whole. The somewhat remarkable resemblance between the diurnal variation for the two elements, first remarked on by J.D. Everett (19), is of interest in connexion with recent theoretical conclusions by J.P. Elster and H.F.K. Geitel and by H. Ebert.
In the potential curves of the diagram the ordinates represent the hourly values expressed—as in Tables II. and III.—as percentages of the mean value for the day. If this be overlooked, a wrong impression may be derived as to the absolute amplitudes of the changes. The Kew curves, for instance, might suggest that the range (maximum less minimum hourly value) was larger in June than in December. In reality the December range was 82, the June only 57 volts; but the mean value of the potential was 243 in December as against 111 in June. So again, in the case of the Paris curves, the absolute value of the diurnal range in summer was much greater for the Eiffel Tower than for the Bureau Central, but the mean voltage was 2150 at the former station and only 134 at the latter.
8. Fourier Coefficients.—Diurnal inequalities such as those of Tables II. and III. and intended to eliminate irregular changes, but they also to some extent eliminate regular changes if the hours of maxima and minima or the character of the diurnal variation alter throughout the year. The alteration that takes place in the regular diurnal inequality throughout the year is best seen by analysing it into a Fourier series of the type
c1 sin(t + a1) + c2 sin(2t + a2) + c3 sin(3t + a3) + c4 sin(4t + a4) + ...
where t denotes time counted from (local) midnight, c1, c2, c3, C4, ... are the amplitudes of the component harmonic waves of periods 24, 12, 8 and 6 hours; a1, a2, a3, a4, are the corresponding phase angles. One hour of time t is counted as 15°, and a delay of one hour in the time of maximum answers to a diminution of 15° in a1, of 30° in a2, and so on. If a1, say, varies much throughput the year, or if the ratios of c2, c3, c4, ... to c1, vary much, then a diurnal inequality derived from a whole year, or from a season composed of several months, represents a mean curve arising from the superposition of a number of curves, which differ in shape and in the positions of their maxima and minima. The result, if considered alone, inevitably leads to an underestimate of the average amplitude of the regular diurnal variation.
It is also desirable to have an idea of the size of the irregular changes which vary from one day to the next. On stormy days, as already mentioned, the irregular changes hardly admit of satisfactory treatment. Even on the quietest days irregular changes are always numerous and often large.
Table IV. aims at giving a summary of the several phenomena for a single station, Kew, on electrically quiet days. The first line gives the mean value of the potential gradient, the second the mean excess of the largest over the smallest hourly value on individual days. The hourly values are derived from smoothed curves, the object being to get the mean ordinate for a 60-minute period. If the actual crests of the excursions had been measured the figures in the second line would have been even larger. The third line gives the range of the regular diurnal inequality, the next four lines the amplitudes of the first four Fourier waves into which the regular diurnal inequality has been analysed. These mean values, ranges and amplitudes are all measured in volts per metre (in the open). The last four lines of Table IV. give the phase angles of the first four Fourier waves.
Table IV.—Absolute Potential Data at Kew (12).
| Jan. | Feb. | March. | April. | May. | June. | July. | Aug. | Sept. | Oct. | Nov. | Dec. | ||
| Mean Potential Gradient | 201 | 224 | 180 | 138 | 123 | 111 | 98 | 114 | 121 | 153 | 200 | 243 | |
| Mean of individual daily ranges | 203 | 218 | 210 | 164 | 143 | 143 | 117 | 129 | 141 | 196 | 186 | 213 | |
| Range in Diurnal inequality | 73 | 94 | 83 | 74 | 71 | 57 | 55 | 60 | 54 | 63 | 52 | 82 | |
| Amplitudes of Fourier waves | c1 | 22 | 22 | 17 | 13 | 18 | 9 | 6 | 6 | 9 | 7 | 14 | 30 |
| c2 | 21 | 33 | 34 | 31 | 22 | 23 | 24 | 26 | 23 | 30 | 17 | 21 | |
| c3 | 7 | 10 | 5 | 5 | 3 | 1 | 3 | 2 | 3 | 6 | 5 | 7 | |
| c4 | 2 | 3 | 5 | 6 | 4 | 1 | 4 | 3 | 4 | 3 | 2 | 3 | |
| ° | ° | ° | ° | ° | ° | ° | ° | ° | ° | ° | ° | ||
| Phase angles of Fourier waves | a1 | 206 | 204 | 123 | 72 | 86 | 79 | 48 | 142 | 154 | 192 | 202 | 208 |
| a1 | 170 | 171 | 186 | 193 | 188 | 183 | 185 | 182 | 199 | 206 | 212 | 175 | |
| a3 | 11 | 9 | 36 | 96 | 100 | 125 | 124 | 107 | 16 | 18 | 38 | 36 | |
| a1 | 235 | 225 | 307 | 314 | 314 | 277 | 293 | 313 | 330 | 288 | 238 | 249 | |
It will be noticed that the difference between the greatest and least hourly values is, in all but three winter months, actually larger than the mean value of the potential gradient for the day; it bears to the range of the regular diurnal inequality a ratio varying from 2.0 in May to 3.6 in November.
At midwinter the 24-hour term is the largest, but near midsummer it is small compared to the 12-hour term. The 24-hour term is very variable both as regards its amplitude and its phase angle (and so its hour of maximum). The 12-hour term is much less variable, especially as regards its phase angle; its amplitude shows distinct maxima near the equinoxes. That the 8-hour and 6-hour waves, though small near midsummer, represent more than mere accidental irregularities, seems a safe inference from the regularity apparent in the annual variation of their phase angles.
Table V.—Fourier Series Amplitudes and Phase Angles.
| Place. | Period. | Winter. | Summer. | ||||||
| c1. | c2. | a1. | a2. | c1. | c2. | a1. | a2. | ||
| ° | ° | ° | ° | ||||||
| Kew | 1862-64 | 0.283 | 0.160 | 184 | 193 | 0.127 | 0.229 | 111 | 179 |
| ” | 1898-1904 | .102 | .103 | 206 | 180 | .079 | .213 | 87 | 186 |
| Bureau Central | 1894-98 | .220 | .104 | 223 | 206 | .130 | .200 | 95 | 197 |
| Eiffel Tower | 1896-98 | .. | .. | .. | .. | .133 | .085 | 216 | 171 |
| Sonnblick (22) | 1902-03 | .. | .. | .. | .. | .208 | .120 | 178 | 145 |
| Karasjok | 1903-04 | .356 | .144 | 189 | 155 | .165 | .093 | 141 | 144 |
| Kremsmünster (23) | 1902 | .280 | .117 | 224 | 194 | .166 | .153 | 241 | 209 |
| Potsdam | 1904 | .269 | .101 | 194 | 185 | .096 | .152 | 343 | 185 |
9. Table V. gives some data for the 24-hour and 12-hour Fourier coefficients, which will serve to illustrate the diversity between different stations. In this table, unlike Table IV., amplitudes are all expressed as decimals of the mean value of the potential gradient for the corresponding season. “Winter” means generally the four midwinter, and “summer” the four midsummer, months; but at Karasjok three, and at Kremsmünster six, months are included in each season. The results for the Sonnblick are derived from a comparatively small number of days in August and September. At Potsdam the data represent the arithmetic means derived from the Fourier analysis for the individual months comprising the season. The 1862-1864 data from Kew—due to J.D. Everett (19)—are based on “all” days; the others, except Karasjok to some extent, represent electrically quiet days. The cause of the large difference between the two sets of data for c1 at Kew is uncertain. The potential gradient is in all cases lower in summer than winter, and thus the reduction in c1 in summer would appear even larger than in Table V. if the results were expressed in absolute measure. At Karasjok and Kremsmünster the seasonal variation in a1 seems comparatively small, but at Potsdam and the Bureau Central it is as large as at Kew. Also, whilst the winter values of a1 are fairly similar at the several stations the summer values are widely different. Except at Karasjok, where the diurnal changes seem somewhat irregular, the relative amplitude of the 12-hour term is considerably greater in summer than in winter. The values of a2 at the various stations differ comparatively little, and show but little seasonal change. Thus the 12-hour term has a much greater uniformity than the 24-hour term. This possesses significance in connexion with the view, supported by A.B. Chauveau (21), F. Exner (24) and others, that the 12-hour term is largely if not entirely a local phenomenon, due to the action of the lower atmospheric strata, and tending to disappear even in summer at high altitudes. Exner attributes the double daily maximum, which is largely a consequence of the 12-hour wave, to a thin layer near the ground, which in the early afternoon absorbs the solar radiation of shortest wave length. This layer he believes specially characteristic of arid dusty regions, while comparatively non-existent in moist climates or where foliage is luxuriant. In support of his theory Exner states that he has found but little trace of the double maximum and minimum in Ceylon and elsewhere. C. Nordmann (25) describes some similar results which he obtained in Algeria during August and September 1905. His station, Philippeville, is close to the shores of the Mediterranean, and sea breezes persisted during the day. The diurnal variation showed only a single maximum and minimum, between 5 and 6 P.M. and 4 and 5 A.M. respectively. So again, a few days’ observations on the top of Mont Blanc (4810 metres) by le Cadet (26) in August and September 1902, showed only a single period, with maximum between 3 and 4 P.M., and minimum about 3 A.M. Chauveau points to the reduction in the 12-hour term as compared to the 24-hour term on the Eiffel Tower, and infers the practical disappearance of the former at no great height. The close approach in the values for c1 in Table V. from the Bureau Central and the Eiffel Tower, and the reduction of c2 at the latter station, are unquestionably significant facts; but the summer value for c2 at Karasjok—a low level station—is nearly as small as that at the Eiffel Tower, and notably smaller than that at the Sonnblick (3100 metres). Again, Kew is surrounded by a large park, not devoid of trees, and hardly the place where Exner’s theory would suggest a large value for c2, and yet the summer value of c2 at Kew is the largest in Table V.
10. Observations on mountain tops generally show high potentials near the ground. This only means that the equipotential surfaces are crowded together, just as they are near the ridge of a house. To ascertain how the increase in the voltage varies as the height in the free atmosphere increases, it is necessary to employ kites or balloons. At small heights Exner (27) has employed captive balloons, provided with a burning fuse, and carrying a wire connected with an electroscope on the ground. He found the gradient nearly uniform for heights up to 30 to 40 metres above the ground. At great heights free balloons seem necessary. The balloon carries two collectors a given vertical distance apart. The potential difference between the two is recorded, and the potential gradient is thus found. Some of the earliest balloon observations made the gradient increase with the height, but such a result is now regarded as abnormal. A balloon may leave the earth with a charge, or become charged through discharge of ballast. These possibilities may not have been sufficiently realized at first. Among the most important balloon observations are those by le Cadet (1) F. Linke (28) and H. Gerdien (29). The following are samples from a number of days’ results, given in le Cadet’s book. h is the height in metres, P the gradient in volts per metre.
| Aug. 9, 1893 { | h | 824 | 830 | 1060 | 1255 | 1290 | 1745 | 1940 | 2080 | 2310 | 2520 |
| P | 37 | 43 | 43 | 41 | 42 | 34 | 25 | 21 | 18 | 16 | |
| Sep. 11, 1897 { | h | 1140 | 1378 | 1630 | 1914 | 237 | 2786 | 3136 | 3364 | 3912 | 4085 |
| P | 43 | 38 | 33 | 25 | 22 | 21 | 19 | 19 | 14 | 13 |
The ground value on the last occasion was 150. From observations during twelve balloon ascents, Linke concludes that below the 1500-metre level there are numerous sources of disturbance, the gradient at any given height varying much from day to day and hour to hour; but at greater heights there is much more uniformity. At heights from 1500 to 6000 metres his observations agreed well with the formula
dV/dh = 34 − 0.006 h,
V denoting the potential, h the height in metres. The formula makes the gradient diminish from 25 volts per metre at 1500 metres height to 10 volts per metre at 4000 metres. Linke’s mean value for dV/dh at the ground was 125. Accepting Linke’s formula, the potential at 4000 metres is 43,750 volts higher than at 1500 metres. If the mean of the gradients observed at the ground and at 1500 metres be taken as an approximation to the mean value of the gradient throughout the lowest 1500 metres of the atmosphere, we find for the potential at 1500 metres level 112,500 volts. Thus at 4000 metres the potential seems of the order of 150,000 volts. Bearing this in mind, one can readily imagine how close together the equipotential surfaces must lie near the summit of a high sharp mountain peak.
11. At most stations a negative potential gradient is exceptional, unless during rain or thunder. During rain the potential is usually but not always negative, and frequent alternations of sign are not uncommon. In some localities, however, negative potential gradient is by no means uncommon, at least at some seasons, in the absence of rain. At Madras, Michie Smith (30) often observed negative potential during bright August and September days. The phenomenon was quite common between 9.30 A.M. and noon during westerly winds, which at Madras are usually very dry and dusty. At Sodankylä, in 1882-1883, K.S. Lemström and F.C. Biese (31) found that out of 255 observed occurrences of negative potential, 106 took place in the absence of rain or snow. The proportion of occurrences of negative potential under a clear sky was much above its average in autumn. At Sodankylä rain or snowfall was often unaccompanied by change of sign in the potential. At the polar station Godthaab (32) in 1882-1883, negative potential seemed sometimes associated with aurora (see Aurora Polaris).
Lenard, Elster and Geitel, and others have found the potential gradient negative near waterfalls, the influence sometimes extending to a considerable distance. Lenard (33) found that when pure water falls upon water the neighbouring air takes a negative charge. Kelvin, Maclean and Gait (34) found the effect greatest in the air near the level of impact. A sensible effect remained, however, after the influence of splashing was eliminated. Kelvin, Maclean and Galt regard this property of falling water as an objection to the use of a water-dropper indoors, though not of practical importance when it is used out of doors.
12. Elster and Geitel (35) have measured the charge carried by raindrops falling into an insulated vessel. Owing to observational difficulties, the exact measure of success attained is a little difficult to gauge, but it seems fairly certain that raindrops usually carry a charge. Elster and Geitel found the sign of the charge often fluctuate repeatedly during a single rain storm, but it seemed more often than not opposite to that of the simultaneous potential gradient. Gerdien has more recently repeated the experiments, employing an apparatus devised by him for the purpose. It has been found by C.T.R. Wilson (36) that a vessel in which freshly fallen rain or snow has been evaporated to dryness shows radioactive properties lasting for a few hours. The results obtained from equal weights of rain and snow seem of the same order.
13. W. Linss (6) found that an insulated conductor charged either positively or negatively lost its charge in the free atmosphere; the potential V after time t being connected with its initial value V0 by a formula of the type V = V0e−at where a is constant. This was confirmed by Elster and Geitel (7), whose form of dissipation apparatus has been employed in most recent work. The percentage of the charge which is dissipated per minute is usually denoted by a+ or a− according to its sign. The mean of a+ and a− is usually denoted by a± or simply by a, while q is employed for the ratio a−/a+. Some observers when giving mean values take Σ(a−/a+) as the mean value of q, while others take Σ(a−)/Σ(a+). The Elster and Geitel apparatus is furnished with a cover, serving to protect the dissipator from the direct action of rain, wind or sunlight. It is usual to observe with this cover on, but some observers, e.g. A. Gockel, have made long series of observations without it. The loss of charge is due to more than one cause, and it is difficult to attribute an absolutely definite meaning even to results obtained with the cover on. Gockel (37) says that the results he obtained without the cover when divided by 3 are fairly comparable with those obtained under the usual conditions; but the appropriate divisor must vary to some extent with the climatic conditions. Thus results obtained for a+ or a− without the cover are of doubtful value for purposes of comparison with those found elsewhere with it on. In the case of q the uncertainty is much less.
Table VI.—Dissipation. Mean Values.
| Place. | Period. | Season. | Observer or Authority. |
a+ | q |
| Karasjok | 1903-4 | Year | Simpson (10) | 3.57 | 1.15 |
| Wolfenbüttel | Year | Elster and Geitel (39) | 1.33 | 1.05 | |
| Potsdam | 1904 | Year | Lüdeling (40) | 1.13 | 1.33 |
| Kremsmüster | 1902 | Year | Zölss (42) | 1.32 | 1.18 |
| ” | 1903 | Year | Zölss (41) | 1.35 | 1.14 |
| Freiburg | Year | Gockel (43) | .. | 1.41 | |
| Innsbruck | 1902 | Czermak (44) | 1.95 | 0.94 | |
| ” | 1905 | Jan. to June | Defant (45) | 1.47 | 1.17 |
| Mattsee (Salzburg) | 1905 | July to Sept. | von Schweidler (46) | .. | 0.99 |
| Seewalchen | 1904 | July to Sept. | von Schweidler (38) | .. | 1.18 |
| Trieste | 1902-3 | Year | Mazelle (47) | 0.58 | 1.09 |
| Misdroy | 1902 | Lüdeling (40) | 1.09 | 1.58 | |
| Swinemünde | 1904 | Aug. and Sept. | Lüdeling (40) | 1.23 | 1.37 |
| Heligoland (sands) | 1903 | Summer | Elster and Geitel (40) | 1.14 | 1.71 |
| Heligoland plateau | ” | ” | Elster and Geitel (40) | 3.07 | 1.50 |
| Juist (Island) | ” | Elster and Geitel (48) | 1.56 | 1.56 | |
| Atlantic and German Ocean | 1904 | August | Boltzmann (49) | 1.83 | 2.69 |
| Arosa (1800 m.) | 1903 | Feb. to April | Saake (50) | 1.79 | 1.22 |
| Rothhorn (2300 m.) | 1903 | September | Gockel (43) | .. | 5.31 |
| Sonnblick (3100 m.) | 1903 | September | Conrad (22) | .. | 1.75 |
| Mont Blanc (4810 m.) | 1902 | September | le Cadet (43) | .. | 10.3 |
Table VI. gives the mean values of a± and q found at various places. The observations were usually confined to a few hours of the day, very commonly between 11 A.M. and 1 P.M., and in absence of information as to the diurnal variation it is impossible to say how much this influences the results. The first eight stations lie inland; that at Seewalchen (38) was, however, adjacent to a large lake. The next five stations are on the coast or on islands. The final four are at high levels. In the cases where the observations were confined to a few months the representative nature of the results is more doubtful.
On mountain summits q tends to be large, i.e. a negative charge is lost much faster than a positive charge. Apparently q has also a tendency to be large near the sea, but this phenomenon is not seen at Trieste. An exactly opposite phenomenon, it may be remarked, is seen near waterfalls, q becoming very small. Only Innsbruck and Mattsee give a mean value of q less than unity. Also, as later observations at Innsbruck give more normal values for q, some doubt may be felt as to the earlier observations there. The result for Mattsee seems less open to doubt, for the observer, von Schweidler, had obtained a normal value for q during the previous year at Seewalchen. Whilst the average q in at least the great majority of stations exceeds unity, individual observations making q less than unity are not rare. Thus in 1902 (51) the percentage of cases in which q fell short of 1 was 30 at Trieste, 33 at Vienna, and 35 at Kremsmünster; at Innsbruck q was less than 1 on 58 days out of 98.
In a long series of observations, individual values of q show usually a wide range. Thus during observations extending over more than a year, q varied from 0.18 to 8.25 at Kremsmünster and from 0.11 to 3.00 at Trieste. The values of a+, a− and a± also show large variations. Thus at Trieste a+ varied from 0.12 to 4.07, and a− from 0.11 to 3.87; at Vienna a+ varied from 0.32 to 7.10, and a− from 0.78 to 5.42; at Kremsmünster a± varied from 0.14 to 5.83.
14. Annual Variation.—When observations are made at irregular hours, or at only one or two fixed hours, it is doubtful how representative they are. Results obtained at noon, for example, probably differ more from the mean value for the 24 hours at one season than at another. Most dissipation results are exposed to considerable uncertainty on these grounds. Also it requires a long series of years to give thoroughly representative results for any element, and few stations possess more than a year or two’s dissipation data. Table VII. gives comparative results for winter (October to March) and summer at a few stations, the value for the season being the arithmetic mean from the individual months composing it. At Karasjok (10), Simpson observed thrice a day; the summer value there is nearly double the winter both for a+ and a−. The Kremsmünster (42) figures show a smaller but still distinct excess in the summer values. At Trieste (47), Mazelle’s data from all days of the year show no decided seasonal change in a+ or a−; but when days on which the wind was high are excluded the summer value is decidedly the higher. At Freiburg (43), q seems decidedly larger in winter than in summer; at Karasjok and Trieste the seasonal effect in q seems small and uncertain.
Table VII.—Dissipation.
| Place | Winter | Summer | ||||||
| a+ | a− | a± | q | a+ | a− | a± | q | |
| Karasjok 1903-1904 | 2.28 | 2.69 | 2.49 | 1.18 | 4.38 | 4.94 | 4.65 | 1.13 |
| Kremsmüster 1903 | 1.14 | 1.30 | 1.22 | 1.14 | 1.38 | 1.56 | 1.47 | 1.12 |
| Freiburg | .. | .. | .. | 1.57 | .. | .. | .. | 1.26 |
| Trieste 1902-1903 | 0.56 | 0.59 | 0.58 | 1.07 | 0.55 | 0.61 | 0.58 | 1.13 |
| Trieste calm days | .. | .. | 0.35 | .. | .. | .. | 0.48 | .. |
15. Diurnal Variation.—P.B. Zölss (41, 42) has published diurnal variation data for Kremsmünster for more than one year, and independently for midsummer (May to August) and midwinter (December to February). His figures show a double daily period in both a+ and a−, the principal maximum occurring about 1 or 2 P.M. The two minima occur, the one from 5 to 7 A.M., the other from 7 to 8 P.M.; they are nearly equal. Taking the figures answering to the whole year, May 1903 to 1904, a+ varied throughout the day from 0.82 to 1.35, and a− from 0.85 to 1.47. At midsummer the extreme hourly values were 0.91 and 1.45 for a+, 0.94 and 1.60 for a−. The corresponding figures at midwinter were 0.65 and 1.19 for a+, 0.61 and 1.43 for a−. Zölss’ data for q show also a double daily period, but the apparent range is small, and the hourly variation is somewhat irregular. At Karasjok, Simpson found a+ and a− both larger between noon and 1 P.M. than between either 8 and 9 A.M. or 6 and 7 P.M. The 6 to 7 P.M. values were in general the smallest, especially in the case of a+; the evening value for q on the average exceeded the values from the two earlier hours by some 7%.
Summer observations on mountains have shown diurnal variations very large and fairly regular, but widely different from those observed at lower levels. On the Rothhorn, Gockel (43) found a+ particularly variable, the mean 7 A.M. value being 4½ times that at 1 P.M. q (taken as Σ(a−/a+) varied from 2.25 at 5 A.M. and 2.52 at 9 P.M. to 7.82 at 3 P.M. and 8.35 at 7 P.M. On the Sonnblick, in early September, V. Conrad (22) found somewhat similar results for q, the principal maximum occurring at 1 P.M., with minima at 9 P.M. and 6 A.M.; the largest hourly value was, however, scarcely double the least. Conrad found a− largest at 4 A.M. and least at 6 P.M., the largest value being double the least; a+ was largest at 5 A.M. and least at 2 P.M., the largest value being fully 2½ times the least. On Mont Blanc, le Cadet (43) found q largest from 1 to 3 P.M., the value at either of these hours being more than double that at 11 A.M. On the Patscherkofel, H. von Ficker and A. Defant (52), observing in December, found q largest from 1 to 2 P.M. and least between 11 A.M. and noon, but the largest value was only 1½ times the least. On mountains much seems to depend on whether there are rising or falling air currents, and results from a single season may not be fairly representative.
16. Dissipation seems largely dependent on meteorological conditions, but the phenomena at different stations vary so much as to suggest that the connexion is largely indirect. At most stations a+ and a− both increase markedly as wind velocity rises. From the observations at Trieste in 1902-1903 E. Mazelle (47) deduced an increase of about 3% in a+ for a rise of 1 km. per hour in wind velocity. The following are some of his figures, the velocity v being in kilometres per hour:—
| v | 0 to 4. | 20 to 24. | 40 to 49. | 60 to 69. |
| a | 0.33 | 0.64 | 1.03 | 1.38 |
| q | 1.13 | 1.19 | 1.00 | 0.96 |
For velocities from 0 to 24 km. per hour q exceeded unity in 74 cases out of 100; but for velocities over 50 km. per hour q exceeded unity in only 40 cases out of 100. Simpson got similar results at Karasjok; the rise in a+ and a− with increased wind velocity seemed, however, larger in winter than in summer. Simpson observed a fall in q for wind velocities exceeding 2 on Beaufort’s scale. On the top of the Sonnblick, Conrad observed a slight increase of a± as the wind velocity increased up to 20 km. per hour, but for greater velocities up to 80 km. per hour no further decided rise was observed.
At Karasjok, treating summer and winter independently, Simpson (10) found a+ and a− both increase in a nearly linear relation with temperature, from below −20° to +15° C. For example, when the temperature was below −20° mean values were 0.76 for a+ and 0.91 for a−; for temperatures between -10° and -5° the corresponding means were 2.45 and 2.82; while for temperatures between +10° and +15° they were 4.68 and 5.23. Simpson found no certain temperature effect on the value of q. At Trieste, from 470 days when the wind velocity did not exceed 20 km. per hour, Mazelle (47) found somewhat analogous results for temperatures from 0° to 30° C.; a−, however, increased faster than a+, i.e. q increased with temperature. When he considered all days irrespective of wind velocity, Mazelle found the influence of temperature obliterated. On the Sonnblick, Conrad (22) found a± increase appreciably as temperature rose up to 4° or 5° C.; but at higher temperatures a decrease set in.
Observations on the Sonnblick agree with those at low-level stations in showing a diminution of dissipation with increase of relative humidity. The decrease is most marked as saturation approaches. At Trieste, for example, for relative humidities between 90 and 100 the mean a± was less than half that for relative humidities under 40. With certain dry winds, notably Föhn winds in Austria and Switzerland, dissipation becomes very high. Thus at Innsbruck Defant (45) found the mean dissipation on days of Föhn fully thrice that on days without Föhn. The increase was largest for a+, there being a fall of about 15% in q. In general, a+ and a− both tend to be less on cloudy than on bright days. At Kiel (53) and Trieste the average value of q is considerably less for wholly overcast days than for bright days. At several stations enjoying a wide prospect the dissipation has been observed to be specially high on days of great visibility when distant mountains can be recognized. It tends on the contrary to be low on days of fog or rain.
The results obtained as to the relation between dissipation and barometric pressure are conflicting. At Kremsmünster, Zölss (42) found dissipation vary with the absolute height of the barometer, a± having a mean value of 1.36 when pressure was below the normal, as against 1.20 on days when pressure was above the normal. He also found a± on the average about 10% larger when pressure was falling than when it was rising. On the Sonnblick, Conrad (22) found dissipation increase decidedly as the absolute barometric pressure was larger, and he found no difference between days of rising and falling barometer. At Trieste, Mazelle (47) found no certain connexion with absolute barometric pressure. Dissipation was above the average when cyclonic conditions prevailed, but this seemed simply a consequence of the increased wind velocity. At Mattsee, E.R. von Schweidler (46) found no connexion between absolute barometric pressure and dissipation, also days of rising and falling pressure gave the same mean. At Kiel, K. Kaehler (53) found a+ and a− both greater with rising than with falling barometer.
V. Conrad and M. Topolansky (54) have found a marked connexion at Vienna between dissipation and ozone. Regular observations were made of both elements. Days were grouped according to the intensity of colouring of ozone papers, 0 representing no visible effect, and 14 the darkest colour reached. The mean values of a+ and a− answering to 12 and 13 on the ozone scale were both about double the corresponding values answering to 0 and 1 on that scale.
17. A charged body in air loses its charge in more than one way. The air, as is now known, has always present in it ions, some carrying a positive and others a negative charge, and those having the opposite sign to the charged body are attracted and tend to discharge it. The rate of loss of charge is thus largely dependent on the extent to which ions are present in the surrounding air. It depends, however, in addition on the natural mobility of the ions, and also on the opportunities for convection. Of late years many observations have been made of the ionic charges in air. The best-known apparatus for the purpose is that devised by Ebert. A cylinder condenser has its inner surface insulated and charged to a high positive or negative potential. Air is drawn by an aspirator between the surfaces, and the ions having the opposite sign to the inner cylinder are deposited on it. The charge given up to the inner cylinder is known from its loss of potential. The volume of air from which the ions have been extracted being known, a measure is obtained of the total charge on the ions, whether positive or negative. The conditions must, of course, be such as to secure that no ions shall escape, otherwise there is an underestimate. I+ is used to denote the charge on positive ions, I- that on negative ions. The unit to which they are ordinarily referred is 1 electrostatic unit of electricity per cubic metre of air. For the ratio of the mean value of I+ to the mean value of I−, the letter Q is employed by Gockel (55), who has made an unusually complete study of ionic charges at Freiburg. Numerous observations were also made by Simpson (10)—thrice a day—at Karasjok, and von Schweidler has made a good many observations about 3 P.M. at Mattsee (46) in 1905, and Seewalchen (38) in 1904. These will suffice to give a general idea of the mean values met with.
| Station. | Authority. | I+ | I− | Q |
| Freiburg | Gockel | 0.34 | 0.24 | 1.41 |
| Karasjok | Simpson | 0.38 | 0.33 | 1.17 |
| Mattsee | von Schweidler | 0.35 | 0.29 | 1.19 |
| Seewalchen | von Schweidler | 0.45 | 0.38 | 1.17 |
Gockel’s mean values of I+ and Q would be reduced to 0.31 and 1.38 respectively if his values for July—which appear abnormal—were omitted. I+ and I− both show a considerable range of values, even at the same place during the same season of the year. Thus at Seewalchen in the course of a month’s observations at 3 P.M., I+ varied from 0.31 to 0.67, and I− from 0.17 to 0.67.
There seems a fairly well marked annual variation in ionic contents, as the following figures will show. Summer and winter represent each six months and the results are arithmetic means of the monthly values.
| Freiburg. | Karasjok. | |||||
| I+ | I− | Q | I+ | I− | Q | |
| Winter | 0.29 | 0.21 | 1.49 | 0.33 | 0.27 | 1.22 |
| Summer | 0.39 | 0.28 | 1.34 | 0.44 | 0.39 | 1.13 |
If the exceptional July values at Freiburg were omitted, the summer values of I+ and Q would become 0.33 and 1.25 respectively.
18. Diurnal Variation.—At Karasjok Simpson found the mean values of I+ and I− throughout the whole year much the same between noon and 1 P.M. as between 8 and 9 A.M. Observations between 6 and 7 P.M. gave means slightly lower than those from the earlier hours, but the difference was only about 5% in I+ and 10% in I−. The evening values of Q were on the whole the largest. At Freiburg, Gockel found I+ and I− decidedly larger in the early afternoon than in either the morning or the late evening hours. His greatest and least mean hourly values and the hours of their occurrence are as follows:—
| Winter. | Summer. | ||||||
| I+ | I− | I+ | I− | ||||
| Max. | Min. | Max. | Min. | Max. | Min. | Max. | Min. |
| 0.333 | 0.193 | 0.242 | 0.130 | 0.430 | 0.244 | 0.333 | 0.192 |
| 2 PM | 7 PM | 2 PM | 8 PM | 4 PM | 9 to | 4 PM | 9 to |
| 10 PM | 10 PM | ||||||
Gockel did not observe between 10 P.M. and 7 A.M.
19. Ionization seems to increase notably as temperature rises. Thus at Karasjok Simpson found for mean values:—
| Temp. less than −20° | −10° to −5° | 10° to 15° |
| I+ = 0.18, I− = 0.36 | I+ = 0.36, I− = 0.30 | I+ = 0.45, I− = 0.43 |
Simpson found no clear influence of temperature on Q. Gockel observed similar effects at Freiburg—though he seems doubtful whether the relationship is direct—but the influence of temperature on I+ seemed reduced when the ground was covered with snow. Gockel found a diminution of ionization with rise of relative humidity. Thus for relative humidities between 40 and 50 mean values were 0.306 for I+ and 0.219 for I−; whilst for relative humidities between 90 and 100 the corresponding means were respectively 0.222 and 0.134. At Karasjok, Simpson found a slight decrease in I− as relative humidity increased, but no certain change in I+. Specially large values of I+ and I− have been observed at high levels in balloon ascents. Thus on the 1st of July 1901, at a height of 2400 metres, H. Gerdien (29) obtained 0.86 for I+ and 1.09 for I−.
20. In 1901 Elster and Geitel found that a radioactive emanation is present in the atmosphere. Their method of measuring the radioactivity is as follows (48): A wire not exceeding 1 mm. in diameter, charged to a negative potential of at least 2000 volts, is supported between insulators in the open, usually at a height of about 2 metres. After two hours’ exposure, it is wrapped round a frame supported in a given position relative to Elster and Geitel’s dissipation apparatus, and the loss of charge is noted. This loss is proportional to the length of the wire. The radioactivity is denoted by A, and A=1 signifies that the potential of the dissipation apparatus fell 1 volt in an hour per metre of wire introduced. The loss of the dissipation body due to the natural ionization of the air is first allowed for. Suppose, for instance, that in the absence of the wire the potential falls from 264 to 255 volts in 15 minutes, whilst when the wire (10 metres long) is introduced it falls from 264 to 201 volts in 10 minutes, then
10A = (254 − 201) × 6 − (264 − 255) × 4 = 342; or A = 34.2.
The values obtained for A seem largely dependent on the station. At Wolfenbüttel, a year’s observations by Elster and Geitel (56) made A vary from 4 to 64, the mean being 20. In the island of Juist, off the Friesland coast, from three weeks’ observations they obtained only 5.2 as the mean. On the other hand, at Altjoch, an Alpine station, from nine days’ observations in July 1903 they obtained a mean of 137, the maximum being 224, and the minimum 92. At Freiburg, from 150 days’ observations near noon in 1903-1904, Gockel (57) obtained a mean of 84, his extreme values being 10 and 420. At Karasjok, observing several times throughout the day for a good many months, Simpson (10) obtained a mean of 93 and a maximum of 432. The same observer from four weeks’ observations at Hammerfest got the considerably lower mean value 58, with a maximum of 252. At this station much lower values were found for A with sea breezes than with land breezes. Observing on the pier at Swinemünde in August and September 1904, Lüdeling (40) obtained a mean value of 34.
Elster and Geitel (58), having found air drawn from the soil highly radioactive, regard ground air as the source of the emanation in the atmosphere, and in this way account for the low values they obtained for A when observing on or near the sea. At Freiburg in winter Gockel (55) found A notably reduced when snow was on the ground, I+ being also reduced. When the ground was covered by snow the mean value of A was only 42, as compared with 81 when there was no snow.
J.C. McLennan (59) observing near the foot of Niagara found A only about one-sixth as large as at Toronto. Similarly at Altjoch, Elster and Geitel (56) found A at the foot of a waterfall only about one-third of its normal value at a distance from the fall.
21. Annual and Diurnal Variations.—At Wolfenbüttel, Elster and Geitel found A vary but little with the season. At Karasjok, on the contrary, Simpson found A much larger at midwinter—notwithstanding the presence of snow—than at midsummer. His mean value for November and December was 129, while his mean for May and June was only 47. He also found a marked diurnal variation, A being considerably greater between 3 and 5 A.M. or 8.30 to 10.30 P.M. than between 10 A.M. and noon, or between 3 and 5 P.M.
At all seasons of the year Simpson found A rise notably with increase of relative humidity. Also, whilst the mere absolute height of the barometer seemed of little, if any, importance, he obtained larger values of A with a falling than with a rising barometer. This last result of course is favourable to Elster and Geitel’s views as to the source of the emanation.
22. For a wire exposed under the conditions observed by Elster and Geitel the emanation seems to be almost entirely derived from radium. Some part, however, seems to be derived from thorium, and H.A. Bumstead (60) finds that with longer exposure of the wire the relative importance of the thorium emanation increases. With three hours’ exposure he found the thorium emanation only from 3 to 5% of the whole, but with 12 hours’ exposure the percentage of thorium emanation rose to about 15. These figures refer to the state of the wire immediately after the exposure; the rate of decay is much more rapid for the radium than for the thorium emanation.
23. The different elements—potential gradient, dissipation, ionization and radioactivity—are clearly not independent of one another. The loss of a charge is naturally largely dependent on the richness of the surrounding air in ions. This is clearly shown by the following results obtained by Simpson (10) at Karasjok for the mean values of a± corresponding to certain groups of values of I±. To eliminate the disturbing influence of wind, different wind strengths are treated separately.
Table VIII.—Mean Values of a±.
| Wind Strength. |
I±0 to 0.1. | 0.1 to 0.2 | 0.2 to 0.3 | 0.3 to 0.4 | 0.4 to 0.5 |
| 0 to 1 | 0.45 | 0.60 | 1.26 | 2.04 | 3.03 |
| 1 to 2 | 0.65 | 1.08 | 1.85 | 2.92 | 3.83 |
| 2 to 3 | .. | .. | 2.70 | 3.88 | 5.33 |
Simspon concluded that for a given wind velocity dissipation is practically a linear function of ionization.
24. Table IX. will give a general idea of the relations of potential gradient to dissipation and ionization.
Table IX.—Potential, Dissipation, Ionization.
| Potential gradients volts per metre. |
q | Karasjok (Simpson (10)). | ||||||
| Kremsmünster (41). | Freiburg (43). | Rothhorn (43). | a+ | a− | I+ | I− | Q | |
| 0 to 50 | .. | 1.12 | .. | .. | .. | .. | .. | .. |
| 50 to 100 | 1.14 | 1.31 | .. | 4.29 | 4.67 | 0.43 | 0.39 | 1.11 |
| 100 to 150 | 1.24 | 1.69 | .. | 3.38 | 3.93 | 0.37 | 0.32 | 1.15 |
| 150 to 200 | 1.48 | 1.84 | .. | 1.85 | 2.58 | 0.36 | 0.28 | 1.28 |
| 200 to 300 | .. | .. | 3.21 | 1.37 | 1.58 | 0.26 | 0.19 | 1.42 |
| 300 to 400 | .. | .. | 4.33 | 0.60 | 0.85 | .. | .. | .. |
| 400 to 500 | .. | .. | 5.46 | .. | .. | .. | .. | .. |
| 500 to 700 | .. | .. | 8.75 | .. | .. | .. | .. | .. |
If we regard the potential gradient near the ground as representing a negative charge on the earth, then if the source of supply of that charge is unaffected the gradient will rise and become high when the operations by which discharge is promoted slacken their activity. A diminution in the number of positive ions would thus naturally be accompanied by a rise in potential gradient. Table IX. associates with rise in potential gradient a reduced number of both positive and negative ions and a diminished rate of dissipation whether of a negative or a positive charge. The rise in q and Q indicates that the diminished rate of dissipation is most marked for positive charges, and that negative ions are even more reduced then positive.
At Kremsmünster Zölss (41) finds a considerable similarity between the diurnal variations in q and in the potential gradient, the hours of the forenoon and afternoon maxima being nearly the same in the two cases.
No distinct relationship has yet been established between potential gradient and radioactivity. At Karasjok Simpson (10) found fairly similar mean values of A for two groups of observations, one confined to cases when the potential gradient exceeded +400 volts, the other confined to cases of negative gradient.
At Freiburg Gockel (55, 57) found that when observations were grouped according to the value of A there appeared a distinct rise in both a− and I+ with increasing A. For instance, when A lay between 100 and 150 the mean value of a- was 1.27 times greater than when A lay between 0 and 50; while when A lay between 120 and 150 the mean value of I+ was 1.53 times larger than when A lay between 0 and 30. These apparent relationships refer to mean values. In individual cases widely different values of a− or I+ are associated with the same value of A.
25. If V be the potential, ρ the density of free electricity at a point in the atmosphere, at a distance r from the earth’s centre, then assuming statical conditions and neglecting variation of V in horizontal directions, we have
r−2(d/dr)(r² dV/dr) + 4πρ = 0.
For practical purposes we may treat r² as constant, and replace d/dr by d/dh, where h is height in centimetres above the ground.
We thus find
ρ = −(1/4π) d²V/dh².
If we take a tube of force 1 sq. cm. in section, and suppose it cut by equipotential surfaces at heights h1 and h2 above the ground, we have for the total charge M included in the specified portion of the tube
4πM = (dV/dh)h1 − (dV/dh)h2.
Taking Linke’s (28) figures as given in § 10, and supposing h1 = 0, h2 = 15 × 104, we find for the charge in the unit tube between the ground and 1500 metres level, remembering that the centimetre is now the unit of length, M = (1/4π) (125 − 25)/100. Taking 1 volt equal 1⁄300 of an electrostatic unit, we find M = 0.000265. Between 1500 and 4000 metres the charge inside the unit tube is much less, only 0.000040. The charge on the earth itself has its surface density given by σ = −(1/4π) × 125 volts per metre, = 0.000331 in e ectrostatic units. Thus, on the view now generally current, in the circumstances answering to Linke’s experiments we have on the ground a charge of −331 × 10−6 C.G.S. units per sq. cm. Of the corresponding positive charge, 265 × 10−6 lies below the 1500 metres level, 40 × 10−6 between this and the 4000 metres level, and only 26 × 10−6 above 4000 metres.
There is a difficulty in reconciling observed values of the ionization with the results obtained from balloon ascents as to the variation of the potential with altitude. According to H. Gerdien (61), near the ground a mean value for d²V/dh² is −(1⁄10) volt/(metre)². From this we deduce for the charge ρ per cubic centimetre (1/4π) × 10−5 (volt/cm²), or 2.7 × 10−9 electrostatic units. But taking, for example, Simpson’s mean values at Karasjok, we have observed
ρ ≡ I+ − I1 = 0.05 × (cm./metre)3 = 5 × 10−8,
and thus (calculated ρ)/(observed ρ) = 0.05 approximately. Gerdien himself makes I+ − I− considerably larger than Simpson, and concludes that the observed value of ρ is from 30 to 50 times that calculated. The presumption is either that d²V/dh² near the ground is much larger numerically than Gerdien supposes, or else that the ordinary instruments for measuring ionization fail to catch some species of ion whose charge is preponderatingly negative.
26. Gerdien (61) has made some calculations as to the probable average value of the vertical electric current in the atmosphere in fine weather. This will be composed of a conduction and a convection current, the latter due to rising or falling air currents carrying ions. He supposes the field near the earth to be 100 volts per metre, or 1⁄300 electrostatic units. For simplicity, he assumes I+ and I− each equal 0.25 × 10−6 electrostatic units. The specific velocities of the ions—i.e. the velocities in unit field—he takes to be 1.3 × 300 for the positive, and 1.6 × 300 for the negative. The positive and negative ions travel in opposite directions, so the total current is (1⁄300)(0.25 × 10−6)(1.3 × 300 + 1.6 × 300), or 73 × 10−8 in electrostatic measure, otherwise 2.4 × 10−16 amperes per sq. cm. As to the convection current, Gerdien supposes—as in § 25—ρ = 2.7 × 10−9 electrostatic units, and on fine days puts the average velocity of rising air currents at 10 cm. per second. This gives a convection current of 2.7 × 10−8 electrostatic units, or about 1⁄27 of the conduction current. For the total current we have approximately 2.5 × 10−16 amperes per sq. cm. This is insignificant compared to the size of the currents which several authorities have calculated from considerations as to terrestrial magnetism (q.v.). Gerdien’s estimate of the convection current is for fine weather conditions. During rainfall, or near clouds or dust layers, the magnitude of this current might well be enormously increased; its direction would naturally vary with climatic conditions.
27. H. Mache (62) thinks that the ionization observed in the atmosphere may be wholly accounted for by the radioactive emanation. If this is true we should have q = αn², where q is the number of ions of one sign made in 1 cc. of air per second by the emanation, α the constant of recombination, and n the number of ions found simultaneously by, say, Ebert’s apparatus. Mache and R. Holfmann, from observations on the amplitude of saturation currents, deduce q = 4 as a mean value. Taking for α Townsend’s value 1.2 × 10−6, Mache finds n = 1800. The charge on an ion being 3.4 × 10−10 Mache deduces for the ionic charge, I+ or I−, per cubic metre 1800 × 3.4 × 10−10 × 106, or 0.6. This is at least of the order observed, which is all that can be expected from a calculation which assumes I+ and I− equal. If, however, Mache’s views were correct, we should expect a much closer connexion between I and A than has actually been observed.
28. C.T.R. Wilson (63) seems disposed to regard the action of rainfall as the most probable source of the negative charge on the earth’s surface. That great separation of positive and negative electricity sometimes takes place during rainfall is undoubted, and the charge brought to the ground seems preponderatingly negative. The difficulty is in accounting for the continuance in extensive fine weather districts of large positive charges in the atmosphere in face of the processes of recombination always in progress. Wilson considers that convection currents in the upper atmosphere would be quite inadequate, but conduction may, he thinks, be sufficient alone. At barometric pressures such as exist between 18 and 36 kilometres above the ground the mobility of the ions varies inversely as the pressure, whilst the coefficient of recombination α varies approximately as the pressure. If the atmosphere at different heights is exposed to ionizing radiation of uniform intensity the rate of production of ions per cc., q, will vary as the pressure. In the steady state the number, n, of ions of either sign per cc. is given by n = √(q/α), and so is independent of the pressure or the height. The conductivity, which varies as the product of n into the mobility, will thus vary inversely as the pressure, and so at 36 kilometres will be one hundred times as large as close to the ground. Dust particles interfere with conduction near the ground, so the relative conductivity in the upper layers may be much greater than that calculated. Wilson supposes that by the fall to the ground of a preponderance of negatively charged rain the air above the shower has a higher positive potential than elsewhere at the same level, thus leading to large conduction currents laterally in the highly conducting upper layers.
29. Thunder.—Trustworthy frequency statistics for an individual station are obtainable only from a long series of observations, while if means are taken from a large area places may be included which differ largely amongst themselves. There is the further complication that in some countries thunder seems to be on the increase. In temperate latitudes, speaking generally, the higher the latitude the fewer the thunderstorms. For instance, for Edinburgh (64) (1771 to 1900) and London (65) (1763 to 1896) R.C. Mossman found the average annual number of thunderstorm days to be respectively 6.4 and 10.7; while at Paris (1873-1893) E. Renou (66) found 27.3 such days. In some tropical stations, at certain seasons of the year, thunder is almost a daily occurrence. At Batavia (18) during the epoch 1867-1895, there were on the average 120 days of thunder in the year.
As an example of a large area throughout which thunder frequency appears fairly uniform, we may take Hungary (67). According to the statistics for 1903, based on several hundred stations, the average number of days of thunder throughout six subdivisions of the country, some wholly plain, others mainly mountainous, varied only from 21.1 to 26.5, the mean for the whole of Hungary being 23.5. The antithesis of this exists in the United States of America. According to A.J. Henry (68) there are three regions of maximum frequency: one in the south-east, with its centre in Florida, has an average of 45 days of thunder in the year; a second including the middle Mississippi valley has an average of 35 days; and a third in the middle Missouri valley has 30. With the exception of a narrow strip along the Canadian frontier, thunderstorm frequency is fairly high over the whole of the United States to the east of the 100th meridian. But to the west of this, except in the Rocky Mountain region where storms are numerous, the frequency steadily diminishes, and along the Pacific coast there are large areas where thunder occurs only once or twice a year.
30. The number of thunderstorm days is probably a less exact measure of the relative intensity of thunderstorms than statistics as to the number of persons killed annually by lightning per million of the population. Table X. gives a number of statistics of this kind. The letter M stands for “Midland.”
Table X.—Deaths by Lightning, per annum, per million Inhabitants.
| Hungary | 7.7 | Upper Missouri and Plains | 15 |
| Netherlands | 2.8 | Rocky Mountains and Plateau | 10 |
| England, N. M. | 1.8 | South Atlantic | 8 |
| ” E. | 1.3 | Central Mississippi | 7 |
| ” S. M. | 1.2 | Upper ” | 7 |
| ” York and W. M. | 1.1 | Ohio Valley | 7 |
| ” N. | 1.0 | Middle Atlantic | 6 |
| Wales | 0.9 | Gulf States | 5 |
| England, S. E. | 0.8 | New England | 4 |
| ” N. W. | 0.7 | Pacific Coast | <1* |
| ” S. W. | 0.6 | North and South Dakota | 20 |
| London | 0.1 | California | 0 |
| * Note in case of Pacific coast, Table X., “<1” means “less than 1.” | |||
The figure for Hungary is based on the seven years 1897-1903; that for the Netherlands, from data by A.J. Monné (69) on the nine years 1882-1890. The English data, due to R. Lawson (70), are from twenty-four years, 1857-1880; those for the United States, due to Henry (68), are for five years, 1896-1900. In comparing these data allowance must be made for the fact that danger from lightning is much greater out of doors than in. Thus in Hungary, in 1902 and 1903, out of 229 persons killed, at least 171 were killed out of doors. Of the 229 only 67 were women, the only assignable explanation being their rarer employment in the fields. Thus, ceteris paribtis, deaths from lightning are much more numerous in a country than in an industrial population. This is well brought out by the low figure for London. It is also shown conspicuously in figures given by Henry. In New York State, where the population is largely industrial, the annual deaths per million are only three, but of the agricultural population eleven. In states such as Wyoming and the Dakotas the population is largely rural, and the deaths by lightning rise in consequence. The frequency and intensity of thunderstorms are unquestionably greater in the Rocky Mountain than in the New England states, but the difference is not so great as the statistics at first sight suggest.
Table XI.—Annual Variation of Thunderstorms.
| Jan. | Feb. | March. | April. | May. | June. | July. | Aug. | Sept. | Oct. | Nov. | Dec. | |
| Ediburgh | 1.8 | 1.4 | 1.4 | 3.8 | 12.3 | 20.8 | 28.2 | 19.1 | 7.0 | 2.3 | 1.1 | 0.8 |
| London | 0.6 | 0.5 | 1.6 | 6.6 | 12.7 | 18.3 | 25.5 | 19.2 | 9.3 | 3.1 | 1.7 | 0.9 |
| Paris | 0.2 | 0.4 | 2.3 | 7.5 | 14.9 | 21.6 | 22.0 | 17.0 | 9.9 | 3.5 | 0.4 | 0.4 |
| Netherlands | 2.2 | 1.8 | 3.7 | 6.5 | 14.0 | 14.7 | 15.6 | 14.7 | 10.3 | 10.1 | 3.8 | 2.5 |
| France | 2.2 | 2.8 | 4.1 | 8.4 | 13.8 | 18.7 | 14.6 | 13.5 | 10.0 | 6.3 | 3.1 | 2.4 |
| Switzerland | 0.2 | 0.3 | 0.5 | 4.9 | 11.9 | 22.9 | 29.9 | 18.0 | 9.8 | 1.1 | 0.3 | 0.2 |
| Hungary (a) | 0.0 | 0.1 | 1.6 | 5.7 | 20.9 | 25.0 | 23.2 | 15.9 | 5.7 | 1.3 | 0.4 | 0.2 |
| Hungary (b) | 0.0 | 0.0 | 1.0 | 3.2 | 11.8 | 20.6 | 30.7 | 25.3 | 6.9 | 0.5 | 0.0 | 0.0 |
| United States | 0.1 | 0.1 | 1.2 | 4.0 | 14.3 | 25.0 | 27.2 | 20.4 | 5.8 | 1.4 | 0.3 | 0.1 |
| Hong-Kong | 0.0 | 2.1 | 4.3 | 8.5 | 12.8 | 23.4 | 14.9 | 21.3 | 10.6 | 2.1 | 0.0 | 0.0 |
| Trevandrum | 3.2 | 3.8 | 13.1 | 20.9 | 18.6 | 4.9 | 1.2 | 3.5 | 2.5 | 12.9 | 12.0 | 3.3 |
| Batavia | 10.4 | 9.2 | 11.1 | 10.5 | 7.9 | 5.5 | 4.3 | 3.8 | 5.4 | 8.8 | 12.2 | 10.9 |
31. Even at the same place thunderstorms vary greatly in intensity and duration. Also the times of beginning and ending are difficult to define exactly, so that several elements of uncertainty exist in data as to the seasonal or diurnal variation. The monthly data in Table XI. are percentages of the total for the year. In most cases the figures are based on the number of days of thunder at a particular station, or at the average station of a country; but the second set for Hungary relates to the number of lightning strokes causing fire, and the figures for the United States relate to deaths by lightning. The data for Edinburgh, due to R.C. Mossman (64), refer to 130 years, 1771 to 1900. The data for London (1763-1896) are also due to Mossman (65); for Paris (1873-1893) to Renou (66); for the Netherlands (1882-1900) to A.J. Monné (69); for France(71) (1886-1899) to Frou and Hann; for Switzerland to K. Hess (72); for Hungary (67) (1896-1903) to L. von Szalay and others; for the United States (1890-1900) to A.J. Henry (68); for Hong-Kong (73) (1894-1903) to W. Doberck. The Trevandrum (74) data (1853-1864) were due originally to A. Broun; the Batavia data (1867-1895) are from the Batavia Observations, vol. xviii.
Most stations in the northern hemisphere have a conspicuous maximum at midsummer with little thunder in winter. Trevandrum (8° 31′ N.) and Batavia (6° 11′ S.), especially the former, show a double maximum and minimum.
Table XII.—Diurnal Variation of Thunderstorms.
| Hour. | 0-2. | 2-4. | 4-6. | 6-8. | 8-10. | 10-12. | 0′-2′. | 2′-4′. | 4′-6′. | 6′-8′. | 8′-10′. | 10′-12′. |
| Finland (76) | 2.3 | 2.0 | 2.2 | 3.0 | 4.6 | 12.1 | 18.9 | 19.2 | 16.1 | 10.1 | 6.1 | 3.4 |
| Edinburgh (64) | 1.7 | 2.0 | 1.4 | 1.7 | 4.7 | 14.2 | 22.4 | 23.7 | 11.9 | 9.2 | 5.1 | 2.0 |
| Belgium (77) | 3.0 | 2.9 | 1.7 | 1.8 | 2.0 | 6.4 | 12.9 | 21.6 | 19.4 | 15.8 | 8.4 | 4.1 |
| Brocken (78) | 1.6 | 2.5 | 1.3 | 1.3 | 4.2 | 3.1 | 12.1 | 28.6 | 22.4 | 10.1 | 7.2 | 5.6 |
| Switzerland (72) | 3.1 | 2.3 | 2.1 | 1.6 | 2.0 | 7.3 | 13.8 | 20.9 | 20.8 | 14.6 | 8.0 | 3.5 |
| Italy (77) | 1.3 | 1.6 | 1.4 | 2.0 | 3.0 | 8.5 | 19.5 | 26.5 | 16.6 | 9.8 | 8.3 | 1.5 |
| Hungary (i.) (67) | 2.1 | 1.9 | 1.9 | 2.1 | 2.9 | 11.5 | 18.1 | 22.0 | 17.9 | 10.7 | 6.2 | 2.8 |
| Hungary (ii.) (67) | 6.9 | 4.2 | 2.3 | 2.0 | 2.0 | 5.0 | 9.9 | 16.9 | 18.2 | 10.7 | 11.7 | 10.0 |
| Hungary (iii.) (75) | 2.3 | 1.9 | 2.0 | 2.4 | 2.7 | 7.9 | 16.1 | 22.1 | 19.1 | 12.7 | 7.6 | 3.2 |
| Hungary (iv.) (75) | 2.6 | 2.2 | 1.9 | 1.9 | 3.6 | 13.3 | 19.9 | 20.7 | 15.2 | 9.2 | 6.2 | 3.3 |
| Trevandrum (74) | 5.6 | 4.9 | 4.3 | 1.3 | 1.4 | 2.0 | 13.3 | 24.5 | 15.9 | 13.3 | 7.6 | 5.9 |
| Agustia (74) | 2.9 | 2.9 | 0.3 | 0.0 | 1.7 | 2.9 | 15.1 | 36.1 | 22.2 | 9.3 | 4.6 | 2.0 |
32. Daily Variation.—The figures in Table XII. are again percentages. They are mostly based on data as to the hour of commencement of thunderstorms. Data as to the hour when storms are most severe would throw the maximum later in the day. This is illustrated by the first two sets of figures for Hungary (67). The first set relate as usual to the hour of commencement, the second to the hours of occurrence of lightning causing fires. Of the two other sets of figures for Hungary (75), (iii.) relates to the central plain, (iv.) to the mountainous regions to north and south of this. The hour of maximum is earlier for the mountains, thunder being more frequent there than in the plains between 8 A.M. and 4 P.M., but less frequent between 2 and 10 P.M. Trevandrum (8° 31′ N., 76° 59′ E., 195 ft. above sea-level) and Agustia (8° 37′ N., 77° 20′ E., 6200 ft. above sea-level) afford a contrast between low ground and high ground in India. In this instance there seems little difference in the hour of maximum, the distinguishing feature being the great concentration of thunderstorm occurrence at Agustia between noon and 6 P.M.
Table XIII.
| Year. | Nether- lands. |
France. | Hungary. | U.S.A. | Year. | Nether- lands. |
France. | Hungary. | U.S.A. |
| 1882 | 98 | .. | 141 | .. | 1893 | 102 | 288 | 233 | 209 |
| 1883 | 117 | .. | 195 | .. | 1894 | 111 | 300 | 333 | 336 |
| 1884 | 95 | .. | 229 | .. | 1895 | 119 | 309 | 280 | 426 |
| 1885 | 93 | .. | 192 | .. | 1896 | 109 | 266 | 299 | 341 |
| 1886 | 102 | 251 | 319 | .. | 1897 | 119 | 297 | 350 | 362 |
| 1887 | 78 | 292 | 236 | .. | 1898 | 95 | 299 | 386 | 367 |
| 1888 | 94 | 286 | 232 | .. | 1899 | 112 | 299 | 368 | 563 |
| 1889 | 126 | 294 | 258 | .. | 1900 | 108 | .. | 401 | 713 |
| 1890 | 93 | 299 | 265 | .. | 1901 | .. | .. | 502 | .. |
| 1891 | 98 | 317 | 302 | 204 | 1902 | .. | .. | 322 | .. |
| 1892 | 86 | 324 | 350 | 251 | 1903 | .. | .. | 256 | .. |
33. Table XIII. gives some data as to the variability of thunder from year to year. The figures for the Netherlands (69) and France (71) are the number of days when thunder occurred somewhere in the country. Its larger area and more varied climate give a much larger number of days of thunder to France. Notwithstanding the proximity of the two countries, there is not much parallelism between the data. The figures for Hungary (67) give the number of lightning strokes causing fire; those for the United States (68) give the number of persons killed by lightning. The conspicuous maximum in 1901 and great drop in 1902 in Hungary are also shown by the statistics as to the number of days of thunder. This number at the average station of the country fell from 38.4 in 1901 to 23.1 in 1902. On the whole, however, the number of destructive lightning strokes and of days of thunder do not show a close parallelism.
Table XIV.
| Decade ending | 1810. | 1820. | 1830. | 1840. | 1850. | 1860. | 1870. | 1880. | 1890. | 1900. |
| Edinburgh | 4.9 | 5.7 | 7.7 | 6.7 | 5.7 | 6.5 | 5.4 | 10.6 | 9.4 | 9.2 |
| London | 9.5 | 8.3 | 11.5 | 11.8 | 10.5 | 11.9 | 9.6 | 15.7 | 13.0 | .. |
| Tilsit | .. | .. | 12.5 | 12.1 | 16.1 | 15.3 | 11.9 | 17.6 | 21.8 | .. |
| Germany, South | .. | .. | .. | .. | .. | 49 | 66 | 91 | 143 | 175 |
| ” West | .. | .. | .. | .. | .. | 92 | 106 | 187 | 244 | 331 |
| ” North | .. | .. | .. | .. | .. | 124 | 135 | 245 | 288 | 352 |
| ” East | .. | .. | .. | .. | .. | 102 | 143 | 186 | 210 | 273 |
| ” Whole | .. | .. | .. | .. | .. | 90 | 116 | 189 | 254 | 318 |
34. Table XIV. deals with the variation of thunder over longer periods. The data for Edinburgh (64) and London (65) due to Mossman, and those for Tilsit, due to C. Kassner (79), represent the average number of days of thunder per annum. The data for Germany, due to O. Steffens (80), represent the average number of houses struck by lightning in a year per million houses; in the first decade only seven years (1854-1860) are really included. Mossman thinks that the apparent increase at Edinburgh and London in the later decades is to some extent at least real. The two sets of figures show some corroborative features, notably the low frequency from 1860 to 1870. The figures for Germany—representing four out of six divisions of that country—are remarkable. In Germany as a whole, out of a million houses the number struck per annum was three and a half times as great in the decade 1890 to 1900 as between 1854 and 1860. Von Bezold (81) in an earlier memoir presented data analogous to Steffens’, seemingly accepting them as representing a true increase in thunderstorm destructiveness. Doubts have, however, been expressed by others—e.g. A. Gockel, Das Gewitter, p. 106—as to the real significance of the figures. Changes in the height or construction of buildings, and a greater readiness to make claims on insurance offices, may be contributory causes.
35. The fact that a considerable number of people sheltering under trees are killed by lightning is generally accepted as a convincing proof of the unwisdom of the proceeding. When there is an option between a tree and an adjacent house, the latter is doubtless the safer choice. But when the option is between sheltering under a tree and remaining in the open it is not so clear. In Hungary (67), during the three years 1901 to 1903, 15% of the total deaths by lightning occurred under trees, as against 57% wholly in the open. In the United States (68) in 1900, only 10% of the deaths where the precise conditions were ascertained occurred under trees, as against 52% in the open. If then the risk under trees exceeds that in the open in Hungary and the United States, at least five or six times as many people must remain in the open as seek shelter under trees. An isolated tree occupying an exposed position is, it should be remembered, much more likely to be struck than the average tree in the midst of a wood. A good deal also depends on the species of tree. A good many years’ data for Lippe (82) in Germany make the liability to lightning stroke as follows—the number of each species being supposed the same:—Oak 57, Fir 39, Pine 5, Beech 1. In Styria, according to K. Prohaska (83), the species most liable to be struck are oaks, poplars and pear trees; beech trees again are exceptionally safe. It should, however, be borne in mind that the apparent differences between different species may be partly a question of height, exposure or proximity to water. A good deal may also depend on the soil. According to Hellmann, as quoted by Henry (82), the liability to lightning stroke in Germany may be put at chalk 1, clay 7, sand 9, loam 22.
36. Numerous attempts have been made to find periodic variations in thunderstorm frequency. Among the periods suggested are the 11-year sun-spot period, or half this (cf. v. Szalay (67)). Ekholm and Arrhenius (84) claim to have established the existence of a tropical lunar period, and a 25.929-day period; while P. Polis (85) considers a synodic lunar period probable. A.B. MacDowall (86) and others have advanced evidence in favour of the view that thunderstorms are most frequent near new moon and fewest near full moon. Much more evidence would be required to produce a general acceptance of any of the above periods.
37. St Elmo’s Fire.—Luminous discharges from masts, lightning conductors, and other pointed objects are not very infrequent, especially during thunderstorms. On the Sonnblick, where the phenomenon is common, Elster and Geitel (87) have found St Elmo’s fire to answer to a discharge sometimes of positive sometimes of negative electricity. The colour and appearance differ in the two cases, red predominating in a positive, blue in a negative discharge. The differences characteristic of the two forms of discharge are described and illustrated in Gockel’s Das Gewitter. Gockel states (l.c. p. 74) that during snowfall the sign is positive or negative according as the flakes are large or are small and powdery. The discharge is not infrequently accompanied by a sizzling sound.
38. Of late years many experiments have been made on the influence of electric fields or currents on plant growth. S. Lemström (88), who was a pioneer in this department, found an electric field highly beneficial in some but not in all cases. Attempts have been made to apply electricity to agriculture on a commercial scale, but the exact measure of success attained remains somewhat doubtful. Lemström believed atmospheric electricity to play an important part in the natural growth of vegetation, and he assigned a special rôle to the needles of fir and pine trees.
Bibliography.—The following abbreviations are here used:—M.Z., Meteorologische Zeitschrift; P.Z., Physikalische Zeitschrift; S., Sitzungsberichte k. Akad. Wiss. Wien, Math. Naturw. Klasse, Theil ii. 2; P.T., “Philosophical Transactions Royal Society of London”; T.M., Terrestrial Magnetism, edited by Dr L.A. Bauer.
Text-books:—(1) G. le Cadet, Étude du champ électrique de l’atmosphère (Paris, 1898); (2) Svante A. Arrhenius, Lehrbuch der kosmischen Physik (Leipzig, 1903); (3) A. Gockel, Das Gewitter (Cologne, 1905).
Lists of original authorities:—(4) F. Exner, M.Z., vol. 17, 1900, p. 529 (especially pp. 542-3); (5) G.C. Simpson, Q.J.R. Met. Soc., vol. 31, 1905, p. 295 (especially pp. 305-6). References in the text:—(6) M.Z., vol. 4, 1887, p. 352; (7) T.M., vol. 4, 1899, p. 213; (8) P.Z., vol. 4, p. 661; (9) M.Z., vol. 23, 1906, p. 114; (10) P.T., vol. 205 A, 1906, p. 61; (11) P.Z., vol. 5, p. 260; (12) C. Chree, P.T., vol. 206 A, p. 299; (13) Annual volumes, Greenwich Magnetical and Meteorological Observations; (14) M.Z., vol. 8, 1891, p. 357; (15) M.Z., vol. 7, 1890, p. 319 and vol. 8, 1891, p. 113; (16) Annual volumes, Annaes do Obs. do Infante D. Luiz; (17) Annual Reports, Central Meteorological Observatory of Japan; (18) Observations made at the Mag. and Met. Obs. at Batavia, vol. 18, 1895; (19) J.D. Everett, P.T., vol. 158, 1868, p. 347; (20) M.Z., vol. 6, 1889, p. 95; (21) A.B. Chauveau, Ann. bureau central météorologique, Paris, année 1900, “Mémoires,” p. C1; (22) V. Conrad, S., 113, p. 1143; (23) P.B. Zölss, P.Z., vol. 5, p. 260; (24) T.M., vol. 7, 1902, p. 89; (25) Revue générale des sciences, 1906, p. 442; (26) T.M., vol. 8, 1903, p. 86. and vol. 9, 1904, p. 147; (27) S., 93, p. 222; (28) M.Z., vol. 22, 1905, p. 237; (29) P.Z., vol. 4, p. 632; (30) Phil. Mag., vol. 20, 1885, p. 456; (31) Expédition polaire finlandaise, vol. 3 (Helsingfors, 1898); (32) A. Paulsen, Bull. de l’Acad. ... de Danemarke, 1894, p. 148; (33) Wied. Ann., vol. 46, 1892, p. 584; (34) P.T., vol. 191 A, p. 187; (35) M.Z., vol. 5, 1888, p. 95; S., 99, p. 421; T.M., vol. 4, 1899, p. 15; (36) Camb. Phil. Soc. Proc., vol. 11, p. 428, and vol. 12, pp. 17 and 85; (37) P.Z., vol. 4, pp. 267 and 873; (38) E.R. v. Schweidler, S., 113, p. 1433; (39) S., 111, July 1902; (40) Veröffentl. des Kg. Preuss. Met. Inst., 1904; (41) P.Z., vol. 5, p. 106; (42) S., 114, p. 198; (43) P.Z., vol. 4, p. 871; (44) P.Z., vol. 4, p. 93; (45) M.Z., vol. 23, 1906, p. 229; (46) S., 114, p. 1705; (47) S., 114, p. 399; (48) P.Z., vol. 4, p. 522; (49) S., 113, p. 1455; (50) P.Z., vol. 4, p. 627; (51) P.Z., vol. 4, p. 90; (52) S., 114, p. 151; (53) M.Z., vol. 23, 1906, p. 253; (54) P.Z., vol. 5, p. 749; (55) M.Z., vol. 23, 1906, pp. 53 and 339; (56) P.Z., vol. 5, p. 11; (57) P.Z., vol. 5, p. 591; (58) T.M., vol. 9, 1904, p. 49; (59) P.Z., vol. 4, p. 295; (60) P.Z., vol. 5, p. 504; (61) T.M., vol. 10, 1905, p. 65; (62) S., 114, p. 1377; (63) Camb. Phil. Soc. Proc., vol. 13, p. 363; (64) Trans. R.S. Edin., vol. 39, p. 63, and vol. 40, p. 484; (65) Q.J.R. Met. Soc., vol. 24, 1898, p. 31; (66) M.Z., vol. 11, 1894, p. 277; (67) Jahrbücher der Konigl. Ung. Reichsanstalt für Met. und Erdmag., vol. 33, 1903, III. Theil with appendix by L. von Szalay; (68) U.S. Dept. of Agriculture, Weather Bureau Bulletin, No. 30, 1901; (69) M.Z., vol. 19, 1902, p. 297; (70) Q.J.R. Met. Soc., vol. 15, 1889, p. 140; (71) M.Z., vol. 20, 1903, p. 227; (72) M.Z., vol. 20, 1903, p. 522; (73) M.Z., vol. 23, 1906, p. 367; (74) M.Z., vol. 22, 1905, p. 175; (75) J. Hegyfoky, M.Z., vol. 20, 1903, p. 218; (76) M.Z., vol. 22, 1905, p. 575; (77) S. Arrhenius, M.Z., vol. 5, 1888, p. 348; (78) G. Hellmann, M.Z., vol. 22, 1905, p. 223; (79) M.Z., vol. 11, 1894, p. 239; (80) M.Z., vol. 23, 1906, p. 468; (81) Berlin Sitz., 1889, No. 16; (82) A.J. Henry, U.S. Dept. of Agriculture Bull., No. 26, 1899; (83) M.Z., vol. 16, 1899, p. 128; (84) K. Sven. Vet. Akad. Hand., Bd. 19, No. 8, Bd. 20, No. 6, Bd. 31, Nos. 2 and 3; (85) M.Z., vol. 11, 1894, p. 230; (86) Nature, vol. 65, 1902, p. 367; (87) M.Z., vol. 8, 1891, p. 321; (88) Brit. Assoc. Report for 1898, p. 808, also Electricity in Agriculture and Horticulture (London, 1904).
1 see Authorities below.


